तीव्रतम अवतरण की विधि

For the optimization algorithm, see Gradient descent.

गणित में, तीव्रतम अवतरण या काठी-बिंदु विधि की विधि एक इंटीग्रल का अनुमान लगाने के लिए लाप्लास की विधि का एक विस्तार है, जहां एक स्थिर बिंदु (सैडल बिंदु) के पास से गुजरने के लिए जटिल विमान में एक समोच्च इंटीग्रल को विकृत किया जाता है, मोटे तौर पर दिशा में तीव्रतम अवतरण या स्थिर चरण। सैडल-पॉइंट सन्निकटन का उपयोग जटिल विमान में इंटीग्रल्स के साथ किया जाता है, जबकि लाप्लास की विधि का उपयोग वास्तविक इंटीग्रल्स के साथ किया जाता है।

अनुमान लगाया जाने वाला अभिन्न अंग अक्सर रूप का होता है

$\int _{C}f(z)e^{\lambda g(z)}\,dz,$

जहां C एक समोच्च है, और λ बड़ा है। सबसे तेज वंश की विधि का एक संस्करण एकीकरण सी के समोच्च को एक नए पथ एकीकरण सी' में विकृत कर देता है ताकि निम्नलिखित स्थितियाँ बनी रहें:

1. C′ व्युत्पन्न g′(z) के एक या अधिक शून्य से होकर गुजरता है,
2. g(z) का काल्पनिक भाग C′ पर स्थिर है।

तीव्रतम अवतरण की विधि सबसे पहले किसके द्वारा प्रकाशित की गई थी? Debye (1909), जिन्होंने बेसेल कार्यों का अनुमान लगाने के लिए इसका उपयोग किया और बताया कि यह अप्रकाशित नोट में हुआ था Riemann (1863)हाइपरज्यामितीय कार्य के बारे में। सबसे तीव्र ढलान के समोच्च में न्यूनतम गुण होता है, देखें Fedoryuk (2001). Siegel (1932) रीमैन के कुछ अन्य अप्रकाशित नोट्स का वर्णन किया, जहां उन्होंने रीमैन-सीगल फॉर्मूला प्राप्त करने के लिए इस विधि का उपयोग किया।

मूल विचार

तीव्रतम अवतरण की विधि प्रपत्र के एक जटिल अभिन्न अंग का अनुमान लगाने की एक विधि है

$$I(\lambda )=\int _{C}f(z)e^{\lambda g(z)}\,\mathrm {d} z$$

बड़े के लिए $\lambda \rightarrow \infty$, कहाँ $f(z)$ और $g(z)$ के विश्लेषणात्मक कार्य हैं $z$. क्योंकि इंटीग्रैंड विश्लेषणात्मक है, रूपरेखा $C$ एक नये स्वरूप में विकृत किया जा सकता है $C'$ अभिन्न को बदले बिना. विशेष रूप से, कोई एक नई रूपरेखा की तलाश करता है जिस पर काल्पनिक भाग हो $g(z)={\text{Re}}[g(z)]+i\,{\text{Im}}[g(z)]$ स्थिर है. तब

$$I(\lambda )=e^{i\lambda {\text{Im}}\{g(z)\}}\int _{C'}f(z)e^{\lambda {\text{Re}}\{g(z)\}}\,\mathrm {d} z,$$

और शेष अभिन्न का अनुमान लाप्लास की विधि जैसी अन्य विधियों से लगाया जा सकता है।

व्युत्पत्ति

विश्लेषणात्मक होने के कारण इस विधि को तीव्रतम अवतरण की विधि कहा जाता है $g(z)$, स्थिर चरण समोच्च तीव्रतम अवरोही समोच्चों के समतुल्य हैं।

अगर $g(z)=X(z)+iY(z)$ का एक विश्लेषणात्मक कार्य है $z=x+iy$, यह कॉची-रीमैन समीकरण को संतुष्ट करता है

$${\frac {\partial X}{\partial x}}={\frac {\partial Y}{\partial y}}\qquad {\text{and}}\qquad {\frac {\partial X}{\partial y}}=-{\frac {\partial Y}{\partial x}}.$$

तब

$${\frac {\partial X}{\partial x}}{\frac {\partial Y}{\partial x}}+{\frac {\partial X}{\partial y}}{\frac {\partial Y}{\partial y}}=\nabla X\cdot \nabla Y=0,$$

इसलिए स्थिर चरण की आकृतियाँ भी तीव्रतम अवतरण की आकृतियाँ हैं।

एक साधारण अनुमान

होने देना  f, S : Cⁿ → C और C ⊂ Cⁿ. अगर

$M=\sup _{x\in C}\Re (S(x))<\infty ,$

कहाँ $\Re (\cdot )$ वास्तविक भाग को दर्शाता है, और एक सकारात्मक वास्तविक संख्या मौजूद है λ₀ ऐसा है कि

$\int _{C}\left|f(x)e^{\lambda _{0}S(x)}\right|dx<\infty ,$

तो निम्नलिखित अनुमान मान्य है:

$\left|\int _{C}f(x)e^{\lambda S(x)}dx\right|\leqslant {\text{const}}\cdot e^{\lambda M},\qquad \forall \lambda \in \mathbb {R} ,\quad \lambda \geqslant \lambda _{0}.$

सरल अनुमान का प्रमाण:

${\begin{aligned}\left|\int _{C}f(x)e^{\lambda S(x)}dx\right|&\leqslant \int _{C}|f(x)|\left|e^{\lambda S(x)}\right|dx\\&\equiv \int _{C}|f(x)|e^{\lambda M}\left|e^{\lambda _{0}(S(x)-M)}e^{(\lambda -\lambda _{0})(S(x)-M)}\right|dx\\&\leqslant \int _{C}|f(x)|e^{\lambda M}\left|e^{\lambda _{0}(S(x)-M)}\right|dx&&\left|e^{(\lambda -\lambda _{0})(S(x)-M)}\right|\leqslant 1\\&=\underbrace {e^{-\lambda _{0}M}\int _{C}\left|f(x)e^{\lambda _{0}S(x)}\right|dx} _{\text{const}}\cdot e^{\lambda M}.\end{aligned}}$

एकल गैर-क्षतिग्रस्त काठी बिंदु का मामला

मूल धारणाएँ और संकेतन

होने देना x एक जटिल हो n-आयामी वेक्टर, और

$S''_{xx}(x)\equiv \left({\frac {\partial ^{2}S(x)}{\partial x_{i}\partial x_{j}}}\right),\qquad 1\leqslant i,\,j\leqslant n,$

किसी फ़ंक्शन के लिए हेस्सियन मैट्रिक्स को निरूपित करें S(x). अगर

${\boldsymbol {\varphi }}(x)=(\varphi _{1}(x),\varphi _{2}(x),\ldots ,\varphi _{k}(x))$

एक वेक्टर फ़ंक्शन है, तो इसके जैकोबियन मैट्रिक्स और निर्धारक को इस प्रकार परिभाषित किया गया है

${\boldsymbol {\varphi }}_{x}'(x)\equiv \left({\frac {\partial \varphi _{i}(x)}{\partial x_{j}}}\right),\qquad 1\leqslant i\leqslant k,\quad 1\leqslant j\leqslant n.$

एक गैर पतित काठी बिंदु, z⁰ ∈ Cⁿ, एक होलोमोर्फिक फ़ंक्शन का S(z) फ़ंक्शन का एक महत्वपूर्ण बिंदु है (यानी, ∇S(z⁰) = 0) जहां फ़ंक्शन के हेसियन मैट्रिक्स में एक गैर-लुप्त होने वाला निर्धारक है (यानी, $\det S''_{zz}(z^{0})\neq 0$).

गैर-अपक्षयी सैडल बिंदु के मामले में इंटीग्रल के एसिम्प्टोटिक्स के निर्माण के लिए निम्नलिखित मुख्य उपकरण है:

कॉम्प्लेक्स मोर्स लेम्मा

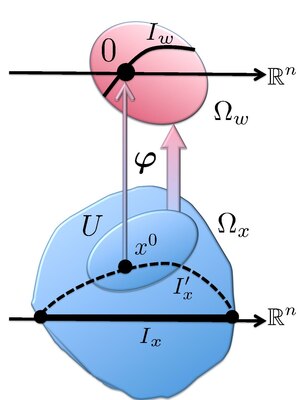
वास्तविक-मूल्यवान कार्यों के लिए मोर्स सिद्धांत#मोर्स लेम्मा निम्नानुसार सामान्यीकृत होता है होलोमोर्फिक फ़ंक्शन के लिए: एक गैर-पतित काठी बिंदु के पास z⁰ एक होलोमोर्फिक फ़ंक्शन का S(z), जिसके संदर्भ में निर्देशांक मौजूद हैं S(z) − S(z⁰) बिल्कुल द्विघात है. इसे सटीक बनाने के लिए आइए Sडोमेन के साथ एक होलोमोर्फिक फ़ंक्शन बनें W ⊂ Cⁿ, और जाने z⁰ में W का एक गैर पतित काठी बिंदु हो S, वह है, ∇S(z⁰) = 0 और $\det S''_{zz}(z^{0})\neq 0$. फिर पड़ोस मौजूद हैं U ⊂ W का z⁰ और V ⊂ Cⁿ का w = 0, और एक वन-टू-वन फ़ंक्शन होलोमोर्फिक फ़ंक्शन φ : V → U साथ φ(0) = z⁰ ऐसा है कि

$\forall w\in V:\qquad S({\boldsymbol {\varphi }}(w))=S(z^{0})+{\frac {1}{2}}\sum _{j=1}^{n}\mu _{j}w_{j}^{2},\quad \det {\boldsymbol {\varphi }}_{w}'(0)=1,$

यहां ही μ_j मैट्रिक्स के आइगेनवैल्यूज़ एवं आइगेनवेक्टर्स हैं $S_{zz}''(z^{0})$.

फ़ाइल:कॉम्प्लेक्स मोर्स लेम्मा चित्रण.पीडीएफ|अंगूठे|केंद्र

Proof of complex Morse lemma

The following proof is a straightforward generalization of the proof of the real Morse Lemma, which can be found in. We begin by demonstrating

Auxiliary statement. Let  f  : Cⁿ → C be holomorphic in a neighborhood of the origin and  f (0) = 0. Then in some neighborhood, there exist functions g_i : Cⁿ → C such that

$$f(z)=\sum _{i=1}^{n}z_{i}g_{i}(z),$$

where each g_i is holomorphic and

$$g_{i}(0)=\left.{\tfrac {\partial f(z)}{\partial z_{i}}}\right|_{z=0}.$$

From the identity

$f(z)=\int _{0}^{1}{\frac {d}{dt}}f\left(tz_{1},\cdots ,tz_{n}\right)dt=\sum _{i=1}^{n}z_{i}\int _{0}^{1}\left.{\frac {\partial f(z)}{\partial z_{i}}}\right|_{z=(tz_{1},\ldots ,tz_{n})}dt,$

we conclude that

$g_{i}(z)=\int _{0}^{1}\left.{\frac {\partial f(z)}{\partial z_{i}}}\right|_{z=(tz_{1},\ldots ,tz_{n})}dt$

and

$g_{i}(0)=\left.{\frac {\partial f(z)}{\partial z_{i}}}\right|_{z=0}.$

Without loss of generality, we translate the origin to z⁰, such that z⁰ = 0 and S(0) = 0. Using the Auxiliary Statement, we have

$S(z)=\sum _{i=1}^{n}z_{i}g_{i}(z).$

Since the origin is a saddle point,

$\left.{\frac {\partial S(z)}{\partial z_{i}}}\right|_{z=0}=g_{i}(0)=0,$

we can also apply the Auxiliary Statement to the functions g_i(z) and obtain

$S(z)=\sum _{i,j=1}^{n}z_{i}z_{j}h_{ij}(z).$

(1)

Recall that an arbitrary matrix A can be represented as a sum of symmetric A^(s) and anti-symmetric A^(a) matrices,

$A_{ij}=A_{ij}^{(s)}+A_{ij}^{(a)},\qquad A_{ij}^{(s)}={\tfrac {1}{2}}\left(A_{ij}+A_{ji}\right),\qquad A_{ij}^{(a)}={\tfrac {1}{2}}\left(A_{ij}-A_{ji}\right).$

The contraction of any symmetric matrix B with an arbitrary matrix A is

$\sum _{i,j}B_{ij}A_{ij}=\sum _{i,j}B_{ij}A_{ij}^{(s)},$

(2)

i.e., the anti-symmetric component of A does not contribute because

$\sum _{i,j}B_{ij}C_{ij}=\sum _{i,j}B_{ji}C_{ji}=-\sum _{i,j}B_{ij}C_{ij}=0.$

Thus, h_ij(z) in equation (1) can be assumed to be symmetric with respect to the interchange of the indices i and j. Note that

$\left.{\frac {\partial ^{2}S(z)}{\partial z_{i}\partial z_{j}}}\right|_{z=0}=2h_{ij}(0);$

hence, det(h_ij(0)) ≠ 0 because the origin is a non-degenerate saddle point.

Let us show by induction that there are local coordinates u = (u₁, ... u_n), z = ψ(u), 0 = ψ(0), such that

$S({\boldsymbol {\psi }}(u))=\sum _{i=1}^{n}u_{i}^{2}.$

(3)

First, assume that there exist local coordinates y = (y₁, ... y_n), z = φ(y), 0 = φ(0), such that

$S({\boldsymbol {\phi }}(y))=y_{1}^{2}+\cdots +y_{r-1}^{2}+\sum _{i,j=r}^{n}y_{i}y_{j}H_{ij}(y),$

(4)

where H_ij is symmetric due to equation (2). By a linear change of the variables (y_r, ... y_n), we can assure that H_rr(0) ≠ 0. From the chain rule, we have

${\frac {\partial ^{2}S({\boldsymbol {\phi }}(y))}{\partial y_{i}\partial y_{j}}}=\sum _{l,k=1}^{n}\left.{\frac {\partial ^{2}S(z)}{\partial z_{k}\partial z_{l}}}\right|_{z={\boldsymbol {\phi }}(y)}{\frac {\partial \phi _{k}}{\partial y_{i}}}{\frac {\partial \phi _{l}}{\partial y_{j}}}+\sum _{k=1}^{n}\left.{\frac {\partial S(z)}{\partial z_{k}}}\right|_{z={\boldsymbol {\phi }}(y)}{\frac {\partial ^{2}\phi _{k}}{\partial y_{i}\partial y_{j}}}$

Therefore:

$S''_{yy}({\boldsymbol {\phi }}(0))={\boldsymbol {\phi }}'_{y}(0)^{T}S''_{zz}(0){\boldsymbol {\phi }}'_{y}(0),\qquad \det {\boldsymbol {\phi }}'_{y}(0)\neq 0;$

whence,

$0\neq \det S''_{yy}({\boldsymbol {\phi }}(0))=2^{r-1}\det \left(2H_{ij}(0)\right).$

The matrix (H_ij(0)) can be recast in the Jordan normal form: (H_ij(0)) = LJL⁻¹, were L gives the desired non-singular linear transformation and the diagonal of J contains non-zero eigenvalues of (H_ij(0)). If H_ij(0) ≠ 0 then, due to continuity of H_ij(y), it must be also non-vanishing in some neighborhood of the origin. Having introduced ${\tilde {H}}_{ij}(y)=H_{ij}(y)/H_{rr}(y)$, we write

$x_{r}={\sqrt {H_{rr}(y)}}\left(y_{r}+\sum _{j=r+1}^{n}y_{j}{\tilde {H}}_{rj}(y)\right),\qquad x_{j}=y_{j},\quad \forall j\neq r.$